MPO
503: Introduction to Physical Oceanography
Notes:
Last
modified: Dec 2014
Vorticity
is an important conceptual tool for understanding the
nature of ocean circulation, because on a rotating
Earth all scales of motion circulate: from inertial
scales (1 km) to eddies at mesoscale (100 km), to
ocean gyres at the large scale (10,000 km). On all
these scales ,the flow has VORTICITY.
As a result, formulating the equations of motion in terms of the conservation of vorticity (similarly to the way we considered conservation of mass, heat and salt) can give some fundamental concepts of the universal qualities of rotating flows.
Mathematically, we define vorticity:
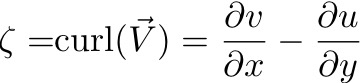
It is the spin about the vertical axis, z. More specifically, it is the shear of the meridional velocity in the zonal direction minus the shear of the zonal velocity in the meridional direction. IF THE FLUID FLOW HAS SHEAR, IT HAS VORTICITY.
How does this work?

This
vorticity, ζ,
that
results from the shear of the local fluid flow is
called RELATIVE vorticity. It is the spin of the fluid
relative to the Earth.
Everything on Earth also has a PLANETARY vorticity, f, which we have seen is twice the local rotation rate of the planet.

Notice that if f is greatest at the poles, is zero at the Equator and is negative in the Southern hemisphere, where latitude is negative by definition.
The sum of the RELATIVE and PLANETARY vorticity is called ABSOLUTE vorticity, ζ + f.
So, lets look at the momentum equations in terms of vorticity and see what we can learn about the flow.
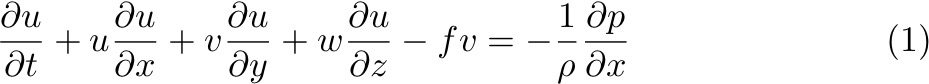
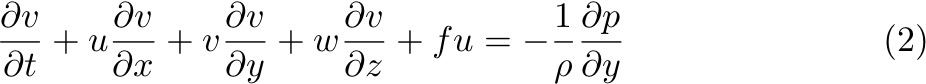
Assume frictional terms are small (away from Ekman layers), and density terms are small. Cross-differentiate these equations as:
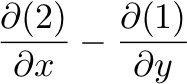
Which leads to:
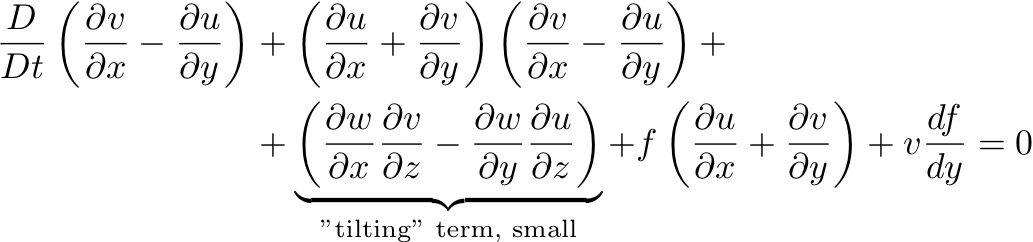
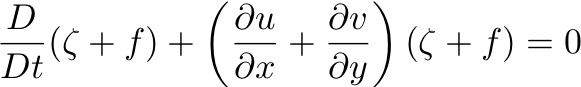
Which is the VORTICITY EQUATION. By using continuity, the divergence term above can be also be written as:
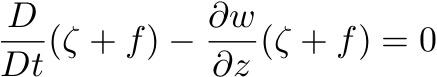
So, considering layer thickness H, within which ρ is a constant and thus velocities u and v are constant, integrate the continuity equation over this thickness:
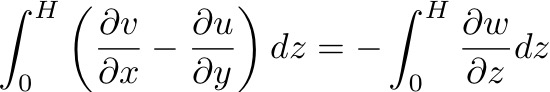
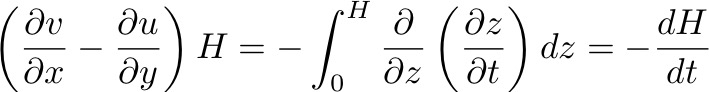
As a result, formulating the equations of motion in terms of the conservation of vorticity (similarly to the way we considered conservation of mass, heat and salt) can give some fundamental concepts of the universal qualities of rotating flows.
Mathematically, we define vorticity:
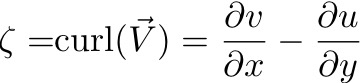
It is the spin about the vertical axis, z. More specifically, it is the shear of the meridional velocity in the zonal direction minus the shear of the zonal velocity in the meridional direction. IF THE FLUID FLOW HAS SHEAR, IT HAS VORTICITY.
How does this work?

Everything on Earth also has a PLANETARY vorticity, f, which we have seen is twice the local rotation rate of the planet.

Notice that if f is greatest at the poles, is zero at the Equator and is negative in the Southern hemisphere, where latitude is negative by definition.
The sum of the RELATIVE and PLANETARY vorticity is called ABSOLUTE vorticity, ζ + f.
So, lets look at the momentum equations in terms of vorticity and see what we can learn about the flow.
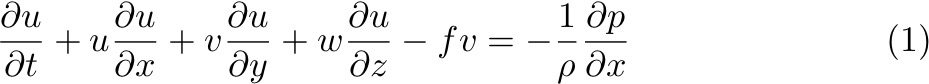
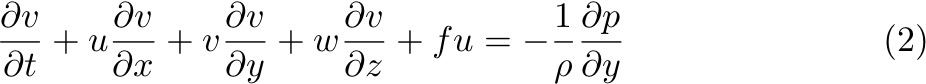
Assume frictional terms are small (away from Ekman layers), and density terms are small. Cross-differentiate these equations as:
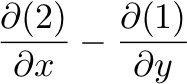
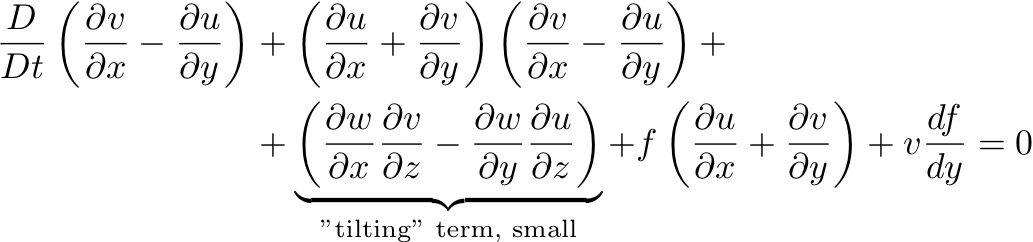
Substitute
for 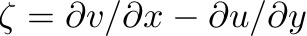 :
:
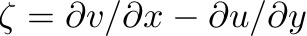 :
: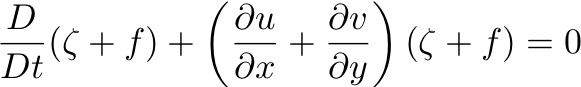
Which is the VORTICITY EQUATION. By using continuity, the divergence term above can be also be written as:
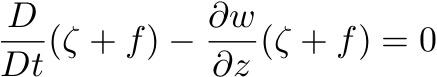
It
is useful to consider this vortex stretching
term in terms of a layer thickness, H - that
is, in terms of a vertical velocity w pumping
water into or out of the layer and hence local
thinning or thickening. This "stretching" of
the layer is going to cause a change to its
spin or
vorticity.
So, considering layer thickness H, within which ρ is a constant and thus velocities u and v are constant, integrate the continuity equation over this thickness:
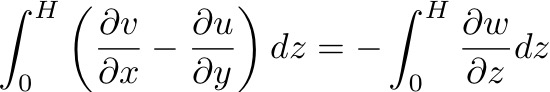
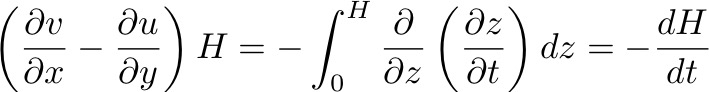
Substituting
for the horizontal divergence in the vorticity
equation we get:
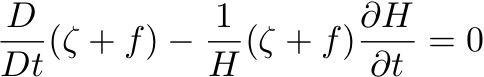
Which can be written:
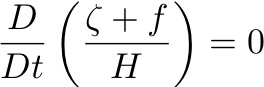
So, in the interior of the ocean, where friction can be considered negligible and hence there is no transfer of momentum between fluid parcels, POTENTIAL VORTICITY is CONSERVED.
The conservation of PV couples changes in relative vorticity to changes in depth, H and latitude. All three interact and balance each other.
1. Consider depth is constant and we change a water parcel's latitude:

So, as a column
of water moves equatorward, | f
| decreases and ζ
must increase. Or, consider a barrel of water
initially at the north pole that is moved quickly
southward where f is smaller - the water will
appear to rotate counterclockwise, since it
acquired positive
ζ.
2. Now consider changes in depth at a constant latitude:

This concept is
analogous with the way that ice skaters change
their spin - by extending their arms outwards they
increase their moment of inertial, which decreases
their rate of spin, and angular momentum is
conserved.
The concept of vorticity and its application to ocean circulation leads to a deeper understanding of the nature of the flows on a rotating Earth. These are a couple of examples:
1. Flows tend to be zonal: In the open ocean, f << ζ and hence f/H = constant. This requires that flows in an ocean of constant depth be zonal (in the absence of an input of vorticity). Specially true close to the Equator, where ∂f/∂y is large.
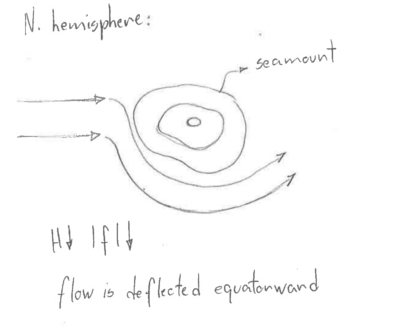
2. Topographic steering: Imagine a seamount or topographic ridge in the deep ocean. Again, f/H is constant - so if H decreases then f must decrease and the flow is turned towards the Equator. If the change in depth is sufficiently large that a reasonable change in latitude cannot compensate, then the flow may be unable to cross the ridge. This is called TOPOGRAPHIC BLOCKING.
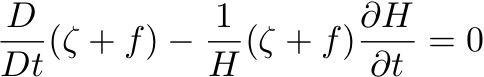
Which can be written:
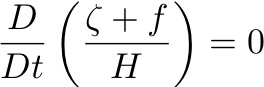
So, in the interior of the ocean, where friction can be considered negligible and hence there is no transfer of momentum between fluid parcels, POTENTIAL VORTICITY is CONSERVED.
The conservation of PV couples changes in relative vorticity to changes in depth, H and latitude. All three interact and balance each other.
1. Consider depth is constant and we change a water parcel's latitude:

2. Now consider changes in depth at a constant latitude:

The concept of vorticity and its application to ocean circulation leads to a deeper understanding of the nature of the flows on a rotating Earth. These are a couple of examples:
1. Flows tend to be zonal: In the open ocean, f << ζ and hence f/H = constant. This requires that flows in an ocean of constant depth be zonal (in the absence of an input of vorticity). Specially true close to the Equator, where ∂f/∂y is large.
2. Topographic steering: Imagine a seamount or topographic ridge in the deep ocean. Again, f/H is constant - so if H decreases then f must decrease and the flow is turned towards the Equator. If the change in depth is sufficiently large that a reasonable change in latitude cannot compensate, then the flow may be unable to cross the ridge. This is called TOPOGRAPHIC BLOCKING.


Lecture
13: Potential Vorticity - the concept of spin/curl/vorticity